Inductor
When a length of wire is formed into a coil, as shown in Figure 1, it becomes a basic inductor. The terms coil and inductor are used interchangeably. Current through the coil produces an electromagnetic field, as illustrated. The magnetic lines of force around each loop (turn) in the winding of the coil effectively add to the lines of force around the adjoining loops, forming a strong electromagnetic field within and around the coil. The net direction of the total electromagnetic field creates a north and a south pole.

To understand the formation of the total electromagnetic field in a coil, consider the interaction of the electromagnetic fields around two adjacent loops. The magnetic lines of force around adjacent loops are each deflected into a single outer path when the loops are brought close together. This effect accurse because the magnetic lines of force are in opposing directions between adjacent loops and therefore cancel out when the loops are close together. The total electromagnetic field for the two loops is depicted in part (b). This effect is additive for many closely adjacent loops in a coil, that is , each additional loop adds to the electromagnetic field. For simplicity only single lines of force are shown, although there are many.
Self Inductance
when there is current through an inductor, an electromagnetic field is established. When the current changes, the electromagnetic field of also changes. An increase in current expands the electromagnetic field, and a decrease in current reduces it. Therefore a changing current produces a changing electromagnetic field around the inductor. In turn, the changing electromagnetic field causes an induced voltage across the coil in a direction to oppose the change in current. This property is called self-inductance but is usually referred to as simply inductance, symbolized by L.
Inductance is measure of a coil's ability to establish an induced voltages as a result of a change in its current, and that induced voltage is in a direction to oppose the change in current.
Unit of Inductance
The henry (H) is the basic unit of inductance. By definition, the inductance of a coil is one henry when current through the coil, changing at the rate of one ampere per second, induces one volt across the coil. In many practical applications, millihenries (mH) and microhenries (µH)are the more common units.
The induced Voltage Depends on L and di / dt
The inductance (L) of a coil and the time rate of change of the current (di/dt) determine the induced voltage (Vind). A change in current causes a change in the electromagnetic field, which, in turn, induces a voltage across the coil, as you know. the induced voltage is directly proportional to L and di/dt, as stated by the following formula.
Vind = L(di/dt)
The formula indicates that the greater the inductance, the greater the induced voltage. Also, it means that the faster the coil current changes (greater di/dt), the greater the induced voltage.
Physical Characteristics of Inductors
The following parameters are important in establishing the inductance of a coil. The ability of the core material, number of turns of wire, core length, and cross section of the core.
Core Material
As discussed above, an inductor is basically a coil of wire that surrounds a magnetic or nonmagnetic material called the core. Examples of magnetic materials are iron,nickel , steel, cobalt, or alloys. These materials have permeability that are hundreds or thousands of times greater than that of a vacuum and are classified as ferromagnetic. A ferromagnetic core provides a better path for the magnetic lines of force and thus permits a stronger magnetic field. Examples of nonmagnetic materials are air, copper, plastic, and glass. the permeabilities of these materials are the same as for a vacuum.
Physical Parameters
The number of turns of wire, the length and the cross-sectional area of the core are factors in setting the value of inductance. The inductance is inversely proportional to the length of the core and directly proportional to the cross-sectional area. Also, the inductance is directly related to the number of turns squared. This relationship is as follows:
L = (N2µA ) / I
where L is the inductance in henry (H), N is the number of turns of wire, µ is the permeability in henry per meter (H/m), A is the cross sectional area in meters squared ant the core length in meters (m).
.
Winding Resistance
When a coil of a certain material, for example, insulated copper wire, that wire has a certain resistance per unit of length. When many turns of wire are used to construct coil, the total resistance may be significant. This inherent resistance is called the dc resistance or the winding resistance (RW).
Although this resistance is distributed along the length of the wire, it effectively appears in series with the inductance of coil, as shown in Figure 5. in many applications, the winding resistance may be small enough to be ignored and the coil considered as an ideal inductor. In other cases, the resistance must be considered.
Figure 5: Inductor Winding Resistance
Winding Capacitance
When two conductors are placed side by side, there is always some capacitance between them. Thus, when many turns of wire are placed close together in a coil. a certain amount of stray capacitance, called winding capacitor is (CW), is natural side effect. In many applications, this winding capacitance is very small and has no significant effect. In other cases, particularly at high frequencies, it may become quite important.
The equivalent circuit of an inductor with both its winding resistance (RW) and its winding capacitance (CW) is shown in Figure 6.
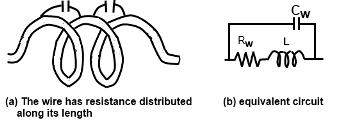
The capacitor effectively acts in parallel. The total of the stray capacitance between each loop of the winding is indicated in a schematic as a capacitance appears in parallel with the coil and its winding resistance, as shown in Figure 6(b).
