Armstrong FM transmitter
Indirect method (phase shift) of modulation
The part of the Armstrong FM transmitter (Armstrong phase modulator) which is expressed in dotted lines describes the principle of operation of an Armstrong phase modulator. It should be noted, first that the output signal from the carrier oscillator is supplied to circuits that perform the task of modulating the carrier signal. The oscillator does not change frequency, as is the case of direct FM. These points out the major advantage of phase modulation (PM), or indirect FM, over direct FM. That is the phase modulator is crystal controlled for frequency.
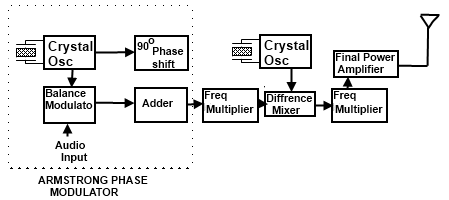
The crystal-controlled carrier oscillator signal is directed to two circuits in parallel. This signal (usually a sine wave) is established as the reference past carrier signal and is assigned a value 0°.
The balanced modulator is an amplitude modulator used to form an envelope of double side-bands and to suppress the carrier signal (DSSC). This requires two input signals, the carrier signal and the modulating message signal. The output of the modulator is connected to the adder circuit; here the 90° phase-delayed carriers signal will be added back to replace the suppressed carrier. The act of delaying the carrier phase by 90° does not change the carrier frequency or its wave-shape. This signal identified as the 90° carrier signal.
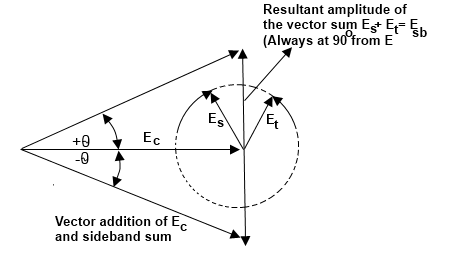
The adder has two input signals, the zero referenced double side-band AM envelope and the 900 carrier signal A vector diagram of the adder output shows the effects o adding, the two input signals. The 90° carrier is labeled E and the vector sum of the two side-bands (Eu and Ec denoted Esh. is shown 90° from Ec.
As the two side-band vectors counter-rotate. Their resultant (Esb) will always be 90° from Ec but will change amplitude and polarity from +Esb to -Esb. The vector addition of Esb and Ec in Figure (b) will form the hypotenuse of the triangle that changes shard through ±0 as the side-band amplitude of Esb changes, from + Esb to - Esb. The hypotenuse represents the output voltages, (Eo) of the adder. As the angle changes from +θ, through 0, to –θ, the length of the hypotenuse (Eo) changes, and since this is the output of the adder, an undesirable amount of amplitude modulation appears at the adder output.
The amount of AM that is acceptable in the PM signal is a matter of how much can be controlled (or eliminated) in later circuits.
Assuming 10% to be the AM limit then,
Emax = Eo
Emin = Ec = 1
So
(% of modulation)(Eo + 1) = (Eo – 1)
Eo (% of modulation) + (% of modulation) = Eo -1
Eo – Eo (% of modulation) = 1 + (% of modulation)
Eo (1 - % of modulation) = 1 + % of modulation.
For 10% AM
So
Eo = 1.222 x Ec
Knowing Eo and Ec, we can find the angle as
Cosθ = 1/1.222
Or θ = ± 34.1o phase shift.
θ = ± 0.6125 rad.
The carrier frequency change at the adder output is a function of the output phase shift and is found by.
fc = ∆θfs (in hertz)
When θ is the phase change in radians and fs is the lowest audio modulating frequency. In most FM radio bands, the lowest audio frequency is 50Hz. Therefore, the carrier frequency change at the adder output is 0.6125 x 50Hz = ± 30Hz since 10% AM represents the upper limit of carrier voltage change, then ± 30Hz is the maximum deviation from the modulator for PM.
The 90° phase shift network does not change the signal frequency because the components and resulting phase change are constant with time. However, the phase of the adder output voltage is in a continual state of change brought about by the cyclical variations of the message signal, and during the time of a phase change, there will also be a frequency change.
In figure. (c). during time (a), the signal has a frequency f1, and is at the zero reference phase. During time (c), the signal has a frequency f1 but has changed phase to θ. During time (b) when the phase is in the process of changing, from 0 to θ. the frequency is less than f1.
